Understanding Factor Pairs: CCSS.4.OA.B.4 worksheets

Welcome, parents and teachers! Today, we’re diving into an important math concept covered by the Common Core State Standards: factor pairs. Specifically, we’ll be looking at standard CCSS.Math.Content.4.OA.B.4, which focuses on factors and multiples for fourth-grade students.
What are Factor Pairs?
Factor pairs are two numbers that, when multiplied together, result in a specific product.
For example, if we’re looking at the number 12, its pair numbers would be:
– 1 and 12
– 2 and 6
– 3 and 4
| Number | Factor pairs |
12 | 1 x 12 = 12 |
| 2 x 6 = 12 | |
| 3 x 4 = 12 |
These pairs all multiply to give us 12. Understanding number pairs helps students grasp the concept of division and multiplication, as well as prime and composite numbers.
Another example:
| Number | Factor pairs |
24 | 1 x 24 = 24 |
| 2 x 12 = 24 | |
| 3 x 8 = 24 | |
| 4 x 6 = 24 | |
| Factor Pairs of 24: 1 and 24, 2 and 12, 3 and 8, 4 and 6 | |
Examples of Factor Pairs:
Let’s explore some common factor number pairs that fourth-grade students typically work with:
- Factor Pairs of 24: 1 and 24, 2 and 12, 3 and 8, 4 and 6
- Factor Pairs of 42: 1 and 42, 2 and 21, 3 and 14, 6 and 7
- Factor Pairs of 36: 1 and 36, 2 and 18, 3 and 12, 4 and 9, 6 and 6
- Factor Pairs of 48: 1 and 48, 2 and 24, 3 and 16, 4 and 12, 6 and 8
- Factor Pairs of 60: 1 and 60, 2 and 30, 3 and 20, 4 and 15, 5 and 12, 6 and 10
- Factor Pairs of 100: 1 and 100, 2 and 50, 4 and 25, 5 and 20, 10 and 10
- Factor Pairs of 18: 1 and 18, 2 and 9, 3 and 6
- Factor Pairs of 30: 1 and 30, 2 and 15, 3 and 10, 5 and 6
It’s important for students to notice patterns as they work with number relationships:
- Every number has 1 and itself as a factor pair.
- If a number is even, it always has 2 as a factor.
- Square numbers (like 36 and 100) have a factor pair where both numbers are the same.
- Some numbers have many factor pairs (like 24, 36, 48, 60), while others have very few (like prime numbers, which only have two factors).
Worksheet resource for practice of factor pairs
To help reinforce this concept, we’re excited to share a valuable worksheet resource from the workybooks library. This worksheet, titled “Build a Factor Tower,” provides an excellent opportunity for students to practice identifying factors and distinguishing between prime and composite numbers.
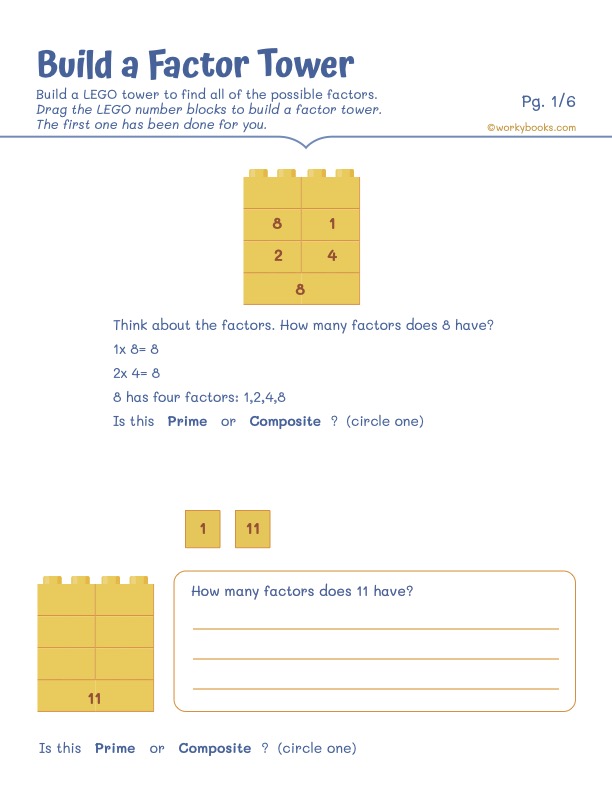
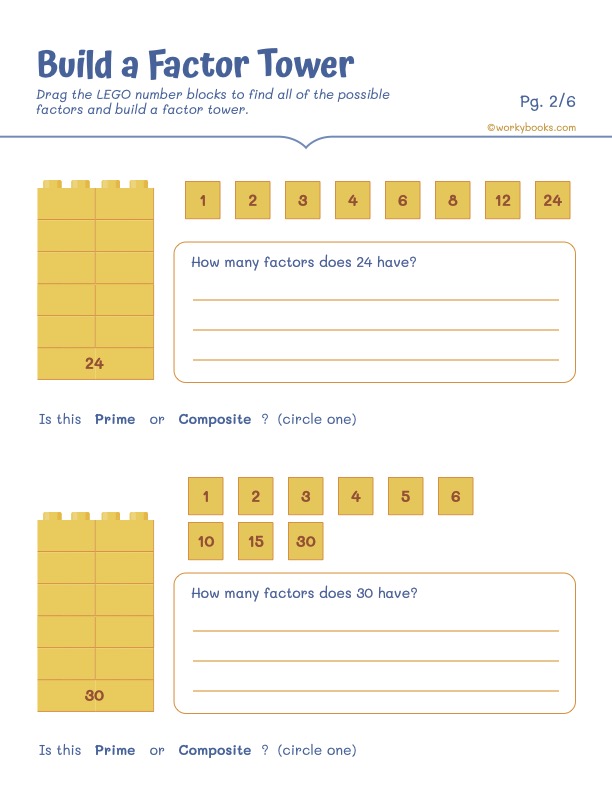
Key features of the 4th grade maths worksheet:
- It covers a range of numbers from 8 to 96, providing ample practice with various number relationships.
- For each number, students must determine:
- How many factors the number has
- Whether the number is prime or composite
- The worksheet includes examples such as 8, 11, 24, 30, 31, 48, 59, 60, 72, 85, and 96, offering a good mix of prime and composite numbers

Prime vs Composite Numbers
As students work with number relationships, they’ll naturally encounter the concepts of prime and composite numbers. Understanding the difference between these two types of numbers is crucial for developing a deeper understanding of factors and multiples.
Prime Numbers
Definition: A prime number is a number that has exactly two factors: 1 and itself.
Examples: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47
Key points:2 is the only even prime number.
1 is neither prime nor composite.
Prime numbers cannot be divided evenly by any number other than 1 and themselves.
Composite Numbers
Definition: A composite number is a number that has more than two factors.
Examples: 4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20, 21, 22, 24, 25, 26, 27, 28, 30
Key points:All even numbers greater than 2 are composite.
The smallest composite number is 4.
Composite numbers can be divided evenly by numbers other than 1 and themselves.
Here is a worksheet for practicing concept of composite numbers.
Understanding factor pairs is a crucial step in developing a strong foundation in mathematics. By using engaging activities like LEGO Factor Towers and supplementing with structured practice using resources like the workybooks worksheet, we can help students visualize these abstract concepts and prepare them for more advanced topics in the future.