Teaching Rectangular Prism in Grade 5 Math

As a 5th grade math teacher, teaching the formulas for volume of rectangular prisms to students can be challenging. However, this foundational geometry standard builds critical spatial reasoning abilities. That’s why incorporating interactive volume activities, models, and visual diagrams into lessons is so valuable. With practical, concrete examples of calculating the cubic units taken up by various rectangular prisms, you can make Standard 5.MD.C.5.A engaging for learners.

In this post, discover key explanations and free worksheet resources.

What is a Rectangular Prism?

A rectangular prism is a three-dimensional geometric shape that has six rectangular faces and opposite sides that are parallel and equal in length. Key features include:

• Six flat sides that are rectangles
• Vertices (corners) formed by three intersecting edges
• Height, width, and depth as dimensions

Common real-world examples students may recognize are boxes, shipping containers, desk drawers, books, and building blocks.

How many edges does a rectangular prism have?

A rectangular prism is a 3D shape with six flat and rectangular sides. Each side meets at three points called vertices (corners). A line connecting two corners or vertices together is called an edge.

If you imagine building a box out of cardboard, each place where the cardboard is folded or connected is an edge. So for example, a cube shaped box would have:

• 4 edges along the bottom
• 4 more edges along the top
• And 4 edges connecting the sides

That’s a total of 12 edges.

Since a rectangular prism is similar to a cube but with differently sized sides, it also has 12 total edges.

To visualize this, imagine a gift box. It has folds or edges along the bottom, the top, and between the sides. Count 4 on bottom + 4 on top + 4 between sides = 12 edges total.

How to find the volume of a rectangular prism?

Finding the volume of a rectangular prism involves multiplying its length x width x height.

The length, width, and height are the three dimensions that make up a rectangular prism. Volume is the total cubic units inside the three-dimensional shape. Here is a solved example, say you have a cuboid shaped box with the following dimensions:

Length = 5 inches

Width = 3 inches

Height = 2 inches

To calculate the volume, use the formula:

Volume = Length x Width x Height

Plug the dimensions into the formula:

V = 5 in x 3 in x 2 in

V = 30 cubic inches

So if a prism has dimensions of 5 inches long, 3 inches wide, and 2 inches high, its volume would be 30 cubic inches. This shows the total space inside the prism shaped box contains 30 cubic units.

The volume formula can be used for any sized rectangular prism if you have its three dimensions – just multiply length x width x height to find the cubic unit volume.

How to find the surface area of a rectangular prism?

The surface area of a rectangular prism is the sum of the areas of all of its sides. There are six rectangular sides on a rectangular prism. 

For example, take a cuboid with these dimensions: 

Length = 5 cm

Width = 3 cm

Height = 2 cm

The formula for finding the area of a rectangle is:  

Area = Length x Width

So for each side it would be:

Side 1 area = 5 cm x 3 cm = 15 sq cm

Side 2 area = 5 cm x 2 cm = 10 sq cm 

Side 3 area = 3 cm x 2 cm = 6 sq cm

Since there are two of each side:

Total Surface Area = 

2 x Side 1 area (15 sq cm)  

+ 2 x Side 2 area  (10 sq cm)

+ 2 x Side 3 area (6 sq cm)

Add the values:

= 30 sq cm + 20 sq cm + 12 sq cm

= 62 square cm

So the total surface area of this prism is 62 square cm.

Here are free 5th grade math worksheets for practicing calculating the volume of a rectangular prism. Aligned to CCSS.MATH.CONTENT.5.MD.C.3 and CCSS.MATH.CONTENT.5.MD.C.3.a

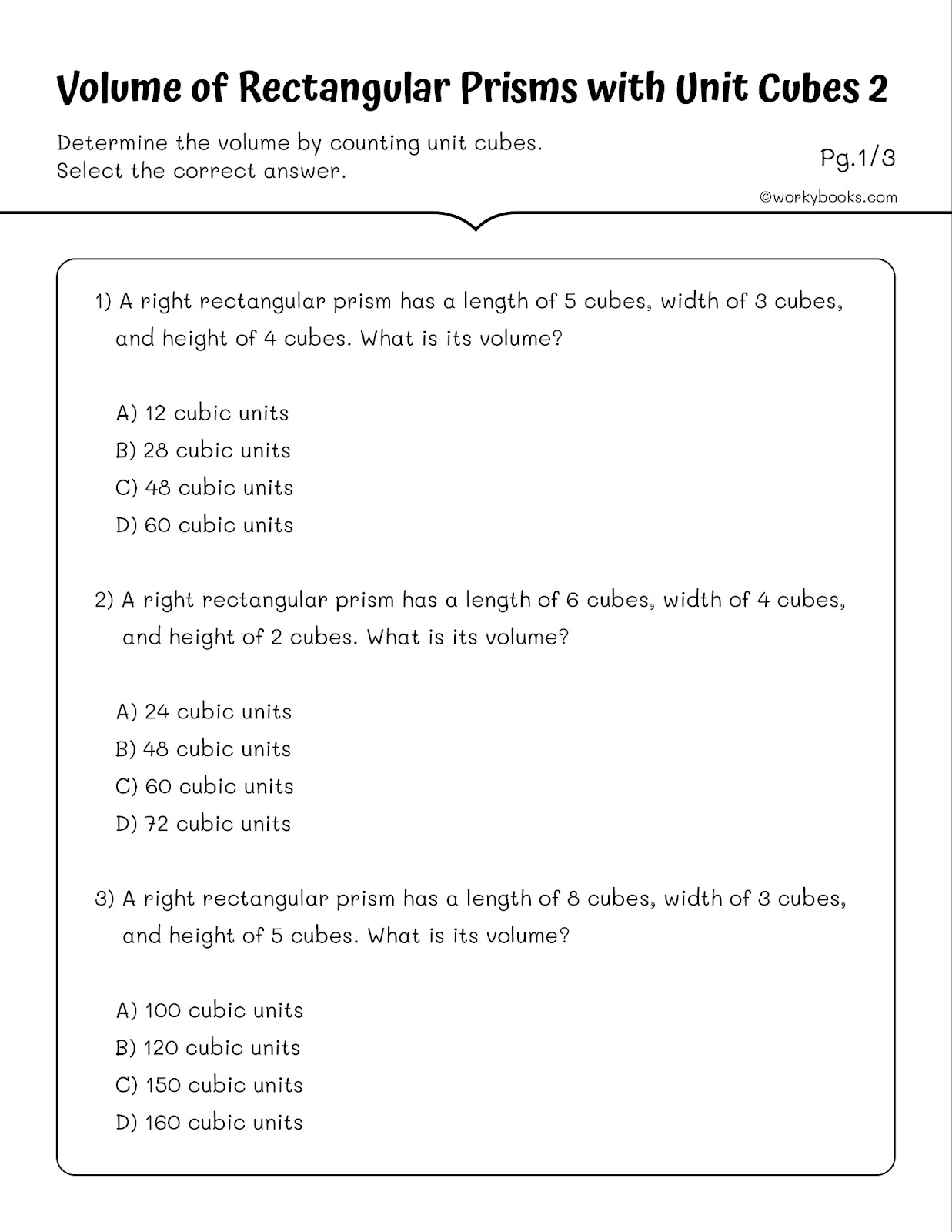
Click here for practice problems on Rectangular Prism with Fractions from Workybooks digital library.